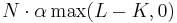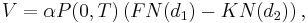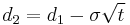Interest rate cap and floor
An interest rate cap is a derivative in which the buyer receives payments at the end of each period in which the interest rate exceeds the agreed strike price. An example of a cap would be an agreement to receive a payment for each month the LIBOR rate exceeds 2.5%.
Similarly an interest rate floor is a derivative contract in which the buyer receives payments at the end of each period in which the interest rate is below the agreed strike price.
Caps and floors can be used to hedge against interest rate fluctuations. For example a borrower who is paying the LIBOR rate on a loan can protect himself against a rise in rates by buying a cap at 2.5%. If the interest rate exceeds 2.5% in a given period the payment received from the derivative can be used to help make the interest payment for that period, thus the interest payments are effectively "capped" at 2.5% from the borrowers point of view.
Contents |
Interest rate cap
An interest rate cap is a derivative in which the buyer receives payments at the end of each period in which the interest rate exceeds the agreed strike price. An example of a cap would be an agreement to receive a payment for each month the LIBOR rate exceeds 2.5%.
The interest rate cap can be analyzed as a series of European call options or caplets which exist for each period the cap agreement is in existence.
In mathematical terms, a caplet payoff on a rate L struck at K is
where N is the notional value exchanged and  is the day count fraction corresponding to the period to which L applies. For example suppose you own a caplet on the six month USD LIBOR rate with an expiry of 1 February 2007 struck at 2.5% with a notional of 1 million dollars. Then if the USD LIBOR rate sets at 3% on 1 February you receive
is the day count fraction corresponding to the period to which L applies. For example suppose you own a caplet on the six month USD LIBOR rate with an expiry of 1 February 2007 struck at 2.5% with a notional of 1 million dollars. Then if the USD LIBOR rate sets at 3% on 1 February you receive
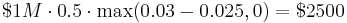
Customarily the payment is made at the end of the rate period, in this case on the 1st of August.
Interest rate floor
An interest rate floor is a series of European put options or floorlets on a specified reference rate, usually LIBOR. The buyer of the floor receives money if on the maturity of any of the floorlets, the reference rate is below the agreed strike price of the floor.
Valuation of interest rate caps
Black model
The simplest and most common valuation of interest rate caplets is via the Black model. Under this model we assume that the underlying rate is distributed log-normally with volatility 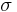 . Under this model, a caplet on a LIBOR expiring at t and paying at T has present value
. Under this model, a caplet on a LIBOR expiring at t and paying at T has present value
where
- P(0,T) is today's discount factor for T
- F is the forward price of the rate. For LIBOR rates this is equal to
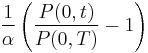
- K is the strike
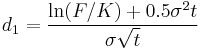
and
Notice that there is a one-to-one mapping between the volatility and the present value of the option. Because all the other terms arising in the equation are indisputable, there is no ambiguity in quoting the price of a caplet simply by quoting its volatility. This is what happens in the market. The volatility is known as the "Black vol" or implied vol.
As a bond put
It can be shown that a cap on a LIBOR from t to T is equivalent to a multiple of a t-expiry put on a T-maturity bond. Thus if we have an interest rate model in which we are able to value bond puts, we can value interest rate caps. Similarly a floor is equivalent to a certain bond call. Several popular short rate models, such as the Hull-White model have this degree of tractability. Thus we can value caps and floors in those models..
What about Collars?
Interest rate collar
…the simultaneous purchase of an interest rate cap and sale of an interest rate floor on the same index for the same maturity and notional principal amount.
- The cap rate is set above the floor rate.
- The objective of the buyer of a collar is to protect against rising interest rates (while agreeing to give up some of the benefit from lower interest rates).
- The purchase of the cap protects against rising rates while the sale of the floor generates premium income.
- A collar creates a band within which the buyer’s effective interest rate fluctuates
And Reverse Collars?
…buying an interest rate floor and simultaneously selling an interest rate cap.
- The objective is to protect the bank from falling interest rates.
- The buyer selects the index rate and matches the maturity and notional principal amounts for the floor and cap.
- Buyers can construct zero cost reverse collars when it is possible to find floor and cap rates with the same premiums that provide an acceptable band.
The size of cap and floor premiums are determined by a wide range of factors
- The relationship between the strike rate and the prevailing 3-month LIBOR
- premiums are highest for in the money options and lower for at the money and out of the money options
- Premiums increase with maturity.
- The option seller must be compensated more for committing to a fixed-rate for a longer period of time.
- Prevailing economic conditions, the shape of the yield curve, and the volatility of interest rates.
- upsloping yield curve—caps will be more expensive than floors.
- the steeper is the slope of the yield curve, ceteris paribus, the greater are the cap premiums.
- floor premiums reveal the opposite relationship.
Valuation of CMS Caps
Caps based on an underlying rateLIBOR (like a Constant Maturity Swap Rate) cannot be valued using simple techniques described above. The methodology for valuation of CMS Caps and Floors can be referenced in more advanced papers.
Implied Volatilities
- An important consideration is cap and floor volatilities. Caps consist of caplets with volatilities dependent on the corresponding forward LIBOR rate. But caps can also be represented by a "flat volatility", so the net of the caplets still comes out to be the same. (15%,20%,....,12%) → (16.5%,16.5%,....,16.5%)
- So one cap can be priced at one vol.
- Another important relationship is that if the fixed swap rate is equal to the strike of the caps and floors, then we have the following put-call parity: Cap-Floor = Swap.
- Caps and floors have the same implied vol too for a given strike.
- Imagine a cap with 20% and floor with 30%. Long cap, short floor gives a swap with no vol. Now, interchange the vols. Cap price goes up, floor price goes down. But the net price of the swap is unchanged. So, if a cap has x vol, floor is forced to have x vol else you have arbitrage.
- A Cap at strike 0% equals the price of a floating leg (just as a call at strike 0 is equivalent to holding a stock) regardless of volatility.
Compare
References
- Damiano Brigo, Fabio Mercurio (2001). Interest Rate Models - Theory and Practice with Smile, Inflation and Credit (2nd ed. 2006 ed.). Springer Verlag. ISBN 978-3-540-22149-4.
External links
- Basic Fixed Income Derivative Hedging - Article on Financial-edu.com.
- Convexity Conundrums by Patrick Hagan
- Martingales and Measures: Black's Model Dr. Jacqueline Henn-Overbeck, University of Basel
- Bond Options, Caps and the Black Model Dr. Milica Cudina, University of Texas at Austin
- Online Caplet And Floorlet Calculator Dr. Shing Hing Man, Thomson Reuters Risk Management
- Introduction to Caps, Floors, Collars and Swaptions